SUPERGROUPS
a computer program for determination of supergroups
of space groups.
S. Ivantchev, E. Kroumova,
G. Madariaga, J. M. Perez-Mato, M. Aroyo, J. Igartua
Departamentos de Física de la Materia Condensada
y Física Aplicada II, Universidad del País Vasco, Apdo 644,
48080 Bilbao, Spain
The crystallographic problem
The problem of the determination of the supergroups of a given
space group is of rather general interest. It is useful for search
of overlooked symmetries in crystal structure determination, or in the
analysis of successive phase transitions, where the introduction of
a hypothetical (supergroup) parent phase can be important. Another
important application is related with the detection of pseudosymmetries
in crystal structures as a method of predicting structural phase
transitions at higher temperatures (Igartua, Aroyo, Perez-Mato, 1996).
There are few papers treating the supergroups of space groups in some
detail (Koch, 1984). The existing listings of minimal supergroups of
space groups are not complete as they provide only a list of those space
groups G which contain a space group H as a maximal non-isomorphic subgroup
(International Tables for Crystallography, vol. A, 1992 (ITA)). This
information is in general not sufficient because it does not include
all the possible supergroups of H isomorphic to G.
The presented program determines
all possible supergroups Gi of H which belong to the space group
type of G.
Method of solution
SUPERGROUPS determines all possible supergroups Gi systematically
inverting the data on maximal subgroups of space groups (International
Tables for Crystallography, vol. A1 (ITA1)). This data has been prepared in
CIF-like format in computer-readable form. Following the procedure based in
normalizers of space groups (Koch 1984, Wondratschek 1996) the program
calculates all supergroups Gi > H, Gi ~ G.
Derivation of supergroups
Supergroup: S; Subgroup: G: G <S
The decomposition of S with respect to G:
S = G + q2G + q3G + ... + qnG
Supergroups S(i) of G, isomorphic to S:
S(i) = ai-1 S ai, S(i) ~ S
Decomposition of S(i) with respect to G:
S(i) = ai-1 G ai +
ai-1 q2 G ai +
ai-1 q3 G ai + ... +
ai-1 qn G ai =
= Gi + q2i Gi +
q3i Gi + ... +
qni Gi,
qkj = aj-1 qk aj
S(i) decomposition with respect to G:
S(i) = G + qi,2 G + qi,3 G + ... + qi,n G
==> TWO sets of supergroups S(i) of G:
- Supergroups conjugated under N(G) (the normalizer of G)
S(i) = ai-1 S ai, ai in N(G)
- Supergroups not conjugated under N(G)
S(i) = ai-1 S ai, ai not in N(G)
[SUPERGROUPS]
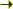 Supergroups
Supergroups
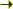 The procedure
The procedure
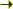 Supergroups
Supergroups
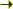 The procedure
The procedure