Bilbao Crystallographic Server Help

Guide to the k-vector figures

The Brillouin zones are projected onto the drawing plane by a clinographic projection. The coordinate axes are designated by k_x, k_y and k_z; the k_z-coordinate axis points upward in the projection plane. The diagrams of the Brillouin zones follow those of CDML [1] in order to facilitate the comparison of the data. The origin O with coefficients (0, 0, 0) coincides always with the centre of the Brillouin zone and is called Γ (indicated as GM in the k-vector tables).

In the Brillouin-zone figures the representation domains of CDML are compared with the asymmetric units of ITA [2]. If the primitive basis of CDML {g₁, g₂, g₃} and the ITA basis {k_x, k_y, k_z} do not coincide then their relations are indicated below the Brillouin-zone figures. A statement if the representation domain of CDML and the asymmetric unit are identical or not is given below the k-vector table. The asymmetric units are often not fully contained in the Brillouin zone but protrude from it, in particular by flagpoles and wings.

In the figures, a point is marked by its label and by a circle filled in with white, if it is listed in the corresponding k-vector table but is not a point of special symmetry. The same designation is used for the auxiliary points that have been added in order to facilitate the comparison between the traditional and the reciprocal-space group descriptions of the k-vector types. Non-coloured parts of the coordinate axes, of the edges of the Brillouin zone or auxiliary lines are displayed by thin solid black lines. Such lines are dashed or omitted if they are not visible, i. e. are hidden by the body of the Brillouin zone or of the asymmetric unit.

The representatives of the orbits of k-vector symmetry points or of symmetry lines, as well as the edges of the representation domains of CDML and of the asymmetric units are brought out in colours.

1. Symmetry points: A representative point of each orbit of symmetry points is designated by a red or cyan filling of the circle with its label also in red or cyan if it belongs to the asymmetric unit or to the representation domain of CDML. If both colours could be used, e. g. if the asymmetric unit coincides with the representation domain, the colour is red. Note that a point is coloured red or cyan only if it is really a symmetry point, i. e. its little co-group is a proper supergroup of the little co-groups of all points in its neighbourhood. Points listed by CDML are not coloured if they are part of a symmetry line or symmetry plane only.
   
   


2. Symmetry lines: Coloured lines are drawn as solid if they are ’visible’, i. e. if they are not hidden by the Brillouin zone or by the asymmetric unit. A hidden symmetry line or edge of the asymmetric unit is not suppressed but is shown as a dashed line. The colour coding of the different lines applied in the Brillouin-zone diagrams is:
   
   

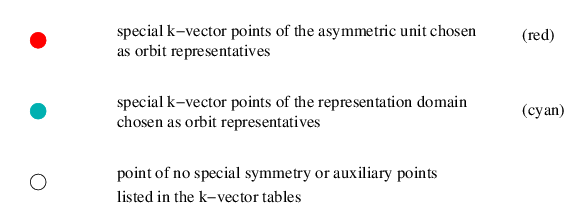
The labels of the special lines shown on the Brillouin-zone figures are always red or cyan irrespectively whether the lines are edges of the representation domain or not. Common edges of an asymmetric unit and a representation domain are coloured pink if they are not symmetry lines simultaneously.

Flagpoles are always coloured red, see, e. g. the line P₁ or PA₁ in the figure of the acute case of the space group R3 (No. 146). Symmetry planes are not distinguished in the figures. However, wings are indicated in the figures and they are always coloured pink, see, e. g. the Brillouin zone figures of C2/c (No. 15) for unique axis c and unique axis b settings.